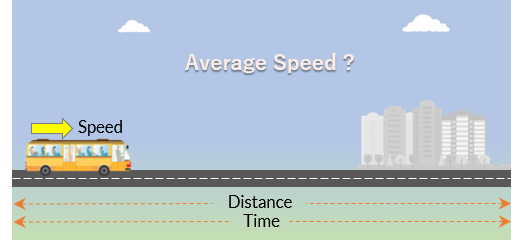
GMAT Word problems on Time, and Speed, and Distance are based on the simple formula of D = S x T, where D is the distance, S is speed and T is time. These GMAT word problems might look easy at first but if your concepts are not clear you will end up cringing in front of the computer screen. You’ll end up wasting valuable time as well as your morale. Therefore, it’s essential you understand how to easily solve Distance Speed Time Problems. This article cites the importance of average speed and its different applications in solving questions on time, speed, and distance. We will learn these concepts through Illustrative Examples.
To get the best learning out of it, you should know some basics such as:
- You are expected to possess a conceptual understanding of the basics of Distance topic
- And the relationship between the three variables; time, speed, and distance – in the form of D = S x T formula
The highlights of this article on GMAT Word Problems on Time, Speed and Distance are as follows
- What will you learn from this article
- The concept of average speed explained with illustrative GMAT word problems
- Calculation of Average Speed when the journey is divided into more than two parts
- Takeaways
What will you learn from this article?
The concept of Average Speed is an important one in the context of GMAT. Hence, the basic objective of this article is to:
- Provide conceptual clarity of the topic, Average Speed
- Solving relevant examples explaining the concepts
- Looking into different learnings and important takeaways
Guillermo scored a Q50 on the GMAT and received an admit for The Wharton School. Take a look at his success story.
For any strategic advice about the GMAT or MBA Admissions, write to us at acethegmat@e-gmat.com. Sign up for a free trial and get unlimited access to concept files, live sessions, and practice questions.
The concept of Average Speed
In theoretical terms, average speed = (Total distance traveled/ Total time taken to travel that distance)
Let’s take an example to have a more detailed explanation of the definition.
Example 1

A bus covers a certain distance at an average speed of 40 mph, while returning the same distance it runs at an average speed of 60 mph. What is the average speed of the bus throughout the journey?
- 20 mph
- 36 mph
- 48 mph
- 50 mph
- 54 mph
Solution
The information in the question can be collated in the following DST table:
| Distance | Speed | Time | |
| Onward Journey | D | 40 mph | – |
| Return Journey | D | 60 mph | – |
A Common Mistake:
While apparently, it may look like the average speed is the arithmetic average of the two speeds given, i.e. (40+60)/2= 50 mph, this is not the correct approach.
If we assume the distance on the onward journey as D, return journey distance will also be D.
- Therefore, the total distance traveled = 2D
Now, the time taken for the onward journey = (D/40) hrs and time taken for the return journey = (D/60) hrs.
- Therefore, the average speed = (Total distance travelled/ total time taken) = 2D/ [(D/40) + (D/60)] = 48 mph
Key Takeaways
As you have observed, although we assumed the value of the unknown distance as D, the final answer doesn’t contain D.
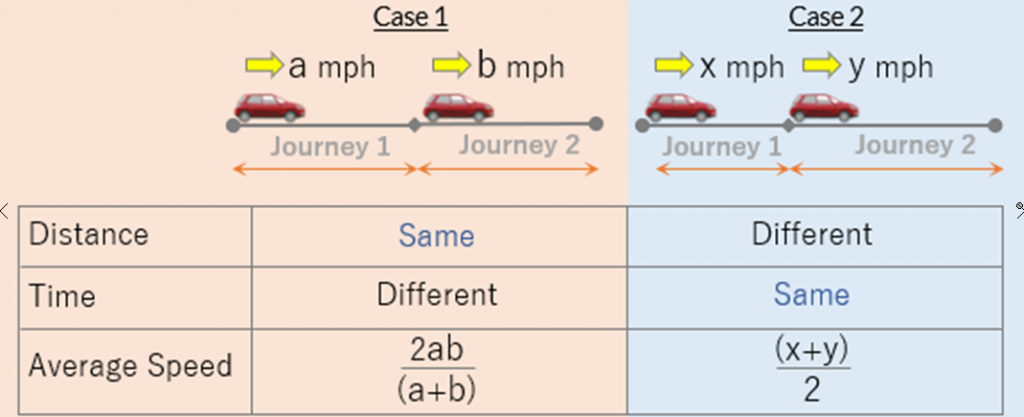
It happens whenever you have the distance as constant and the journey is divided into two equal parts. This can be generalized as follows:
The generalized formula:
Let us assume that a journey has two equidistant part, D each, covered at an average speed of a and b.
Time taken to complete the first half of the journey = D/a
Time taken to complete the second half of the journey = D/b
Therefore, the overall average speed of the journey = 2D/ [(D/40) + (D/60)] = 2ab/a+b
The above specific case shows the example when the journey is divided into two equal parts.
Let’s take another example to understand the application of the above takeaway.
BTW, do you know how to identify a regular polygon? Read our article on Polygons to know more.
Example 2
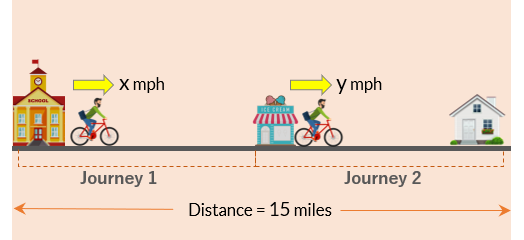
Joey covered his journey of 15 miles in two parts. He covered the 1st part of his journey at x mph and the second part in y mph. What is Joey’s average speed throughout the whole journey?
Statement 1: x = 2.5, y = 5
Statement 2: Distance covered in both parts of the journey is the same
Solution
In this question it is given that:
- Joey covered a journey of total distance 15 miles in two parts
- Speed in the 1st part of the journey = x mph
- Speed in the 2nd part of the journey = y mph
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | D1 | x | |
| 2nd part of the journey | D2 | y | |
| Total | D1 + D2 = 15 |
As we need to find out the average speed of Joey’s whole journey, we need to know two things:
- The total distance, which is already provided as 15 miles
- The total time taken to cover that distance, which is the sum of the individual times taken to cover the 1st and 2nd part separately
Analyzing Statement 1:
Now, analyzing Statement 1 independently, it says x = 2.5 mph and y = 5 mph.
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 2.5 | ||
| 2nd part of the journey | 5 | ||
| Total | 15 |
- Considering this statement only, we get the information about the individual speeds only
- But this does not provide any information about the distances covered in each individual part of the journey – so, we cannot calculate the time taken for the parts of the journey
Hence, Statement 1 is not sufficient to answer the question.
Analyzing Statement 2:
Analyzing Statement 2 independently, it says the distance covered in both parts of the journey is the same.
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 7.5 | x | |
| 2nd part of the journey | 7.5 | y | |
| Total | 15 |
- It means each of the individual parts of the journey is of 7.5 miles.
- But this does not provide any information about the speeds in each individual part of the journey – so, we cannot calculate the time taken for the parts of the journey
Hence, Statement 2 is not sufficient to answer the question.
Combining Both Statements:
Now, combining the information from both the statements, we have:
- Individual speeds in each part, x = 2.5 mph and y = 5 mph
- Distance in each part of the journey = 7.5 miles
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 7.5 | 2.5 | |
| 2nd part of the journey | 7.5 | 5 | |
| Total | 15 |
Considering both together, we can calculate the time taken for individual parts of the journey, and hence the total journey time. From that, we can calculate the average speed.
As we are getting the answer by combining both the statements, correct answer choice is option C.
Note:-
Although for the question you don’t need to calculate the final value, the average speed here will be as follows:
Average Speed = (Total distance traveled)/(Total time taken to travel that distance)
= 15/ [(7.5/2.5) + (7.5/5)] = 15/4.5 = 3.33 mph
Because the distance is divided into two equal parts, we can also calculate the average speeds as
= (2×2.5×5)/(2.5+5) = 3.33 mph [application of the 2ab/a+b formula]
The devil is in the details! Planning out the finer details of your study schedule takes you one step closer to your target GMAT score. Learn the other benefits of a well defined study plan in this article.
Save 60+ hours of GMAT preparation by crafting a well-defined study plan in just 3 steps:
Let us take another example to solidify our understanding and then move to the next topic.
Example 3
Mike started his road trip on his bike and moved at a constant speed of 50 mph. After completing p% of his total journey, his bike started malfunctioning, and therefore, he had to complete his journey at half of his normal speed. What is the average of Mike’s whole journey, in terms of p?
Solution
In this question, first, we can consolidate all the given information.
- Here Mike’s journey is getting divided into two parts –
- 1st part is p% of total journey distance, completed at 50 mph
- 2nd or the remaining part of the journey, completed at 25 mph
We need to find out the average speed of Mike throughout the whole journey.
| Distance | Speed | Time | |
| Before bike malfunction | p % of total journey distance | 50 mph | – |
| After bike malfunction | (100-p) % of the total journey distance | 25 mph | – |
Now you can see that the total distance is not mentioned here. If we assume the total distance to be D, then
1st part of the journey = p% of D = pD/100, and remaining part of the journey = (D-(pD/100))
As we have the individual distances and speeds for every segment of the journey, we can now calculate the time taken for each segment as follows:
Time taken to complete the 1st part of the journey = (pD/100)/50 hrs = pD/5000 hrs
And, time taken to complete the 2nd part of the journey = [(D-pD)/100]/25 hrs = (100D-pD) /2500 hrs
Now that we know the total distance and total time for the journey, we can calculate the average speed of the whole journey as follows:
Average Speed = D/ [(pD/5000) + (100D-pD)/2500] = 5000/ (200-p)
Key Takeaways
- Although we assumed the value of the total distance as D, the final answer is independent of D. This is happening because the distance remains constant and does not influence the average speed.
- Although the journey is taking place in two separate parts, the overall journey distance remains constant. In cases like this, one can assume any constant value as the total distance for the ease of calculation. For example, in this case, if the distance is assumed to be 100 in place of D, the overall simplification process becomes very easy. Check the following:
As the total distance is assumed to be 100, then
- 1st part of the journey = p% of 100 = p, and remaining part of the journey = (100 – p)
As we have the individual distances and speeds for every segment of the journey, we can now calculate the time taken for each segment as follows:
Time taken to complete the 1st part of the journey = p/50 hrs
And, time taken to complete the 2nd part of the journey = (100-p)/25 hrs
Now that we know the total distance and total time for the journey, we can calculate the average speed of the whole journey as follows:
Average speed = 100/(p/50) + (100-p)/25 = 5000/ (200-p)
Example 4
On a certain day, Rosita started driving towards Stevie’s place at a speed of 45 mph. After moving for 2 hours, she realized that she needed to reach Stevie’s place in another 2 hours only. Therefore, she increased her speed to 65 mph and reached Stevie’s place exactly on time. What is the average speed of her journey?
Solution
In this question, the journey has happened in two parts.
- In the 1st part, Rosita moved at 45 mph for 2 hrs. Therefore, the distance covered = (45 × 2) = 90 miles
- In the 2nd part, Rosita moved at 65 mph for 2 hrs. Therefore, the distance covered = (65 × 2) = 130 miles
| Distance (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 45 x 2 = 90 | 45 | 2 |
| 2nd part of the journey | 65 x 2 = 130 | 65 | 2 |
Total distance travelled by Rosita = (90 + 130) miles = 220 miles
Total time taken to travel that distance = (2 + 2) hrs = 4 hrs
Hence, the average speed of the journey = Total distance travelled/ Total time taken = 220/4 mph = 55 mph
Key Takeaways
If looked closely, one can see that the average speed, in this case, is the arithmetic average of the two given speeds. Let us see the logic behind that:
Assume a scenario when a moving body travels at a speed of x mph for t hrs and at a speed of y mph for next ‘t’ hrs
- In the 1st t hours, the distance covered = xt miles
- In the next t hours, the distance covered = yt miles
- The total distance covered = (xt + yt) miles = t (x + y) miles
- Total time taken to cover the distance = (t + t) hrs = 2t hrs
Therefore, the average speed = Total distance travelled/ Total time taken to travel that distance = t(x+y)/2t = (x+y)/2 mph
- which is essentially the numerical average of two speeds given
Hence, we can say if the journey is divided into two parts, in which every part is traveled for the same amount of time, the average speed of the journey is the numerical average of the two individual speeds.
This logic can also be generalized for journeys which are divided into more than two parts, and the time taken to cover each part is same.
Let’s say we are considering a scenario where the journey is divided into 3 parts, with the speeds in the three parts are x, y, and z respectively and the time taken to cover each part of the journey is same.
- In such a scenario, we can say the average speed of the whole journey is nothing but the average or arithmetic mean of the three individual speeds, i.e. x+y+z/3
The same concept can also be generalized for journeys which are divided into multiple parts, with each part takes the same time to cover.
Want to boost your Quant score but no able to define the path to your Target Score? Attend our Quant Workshop and get personalised feedback from Subject Matter Experts which will help you hit your Target GMAT Scores.
Example 5
A bus covers a certain distance at an average speed of 100 mph without any stoppages. While returning the same journey the bus covers the distance at an average speed of 60 mph with stoppages. What is the average stoppage time per hour taken by the bus?
Solution
This question can be solved using multiple methods:
Method 1:
Let’s assume the total journey distance in either direction is D
Therefore, time taken for the onward journey = D/100 hrs
And time taken for the return journey = D/60 hrs
The difference in journey time indicates the stoppage time, which is (D/60)-(D/100) = D/150 hrs
In the journey of D/60 hrs, stoppage time is D/150 hrs
Therefore, the average stoppage time per hour = (D/150)/ (D/60) hrs = 60/150 hrs = 2/5 hrs = 24 minutes
Method 2:
Let’s assume the distance to be 300 miles (LCM of 100 and 60).
Therefore, time for the onward journey = 300/100 = 3 hrs
And time for the return journey = 300/60 = 5 hrs
The extra time indicates the stoppage time, which is (5-3) = 2 hrs
In the journey of 5 hrs, stoppage time is 2 hrs, therefore, average stoppage time per hour = 2/5 hrs = 24 minutes
Method 3:
As the decrease in speed is happening due to stoppages, that decrease would have been absent if there were no stoppages. Here the decrease in speed is (100 – 60) = 40 miles per hour.
Now, when the bus was running without any stoppages, the average speed was 100 mph. At this speed, the bus could have completed 40 miles distance in a time of 40/100 hrs or 24 minutes, which is effectively the stoppage time.
On a similar note, if the decrease in speed was 80 miles per hour, then the bus would have completed that 80 miles distance in a time of 80/100 hrs or 48 minutes.
Key Takeaway
Although the distance remains the same in both onward and return journey, the average speed decreases because of the increase in the total journey time due to stoppages.
Generic case: Calculation of Average Speed when the journey is divided into more than two parts
Till now we have mostly taken up those examples where the average speed is calculated in the journeys divided into two parts. However, the concept of average speed is applicable to cases where the journey is divided into more than 2 parts. Let’s consider the following example:
Example 6
A train covered first 150 miles of its journey at an average speed of 50 mph. After that, it met with a small accident and its speed decreases to ‘a’ mph. It continued its journey for 4 hrs and then its speed decreased further to 20 mph. Running at this speed, the train completed the journey in total 12 hrs. Find the value of ‘a’, if the average speed of the whole journey is 33.33 mph.
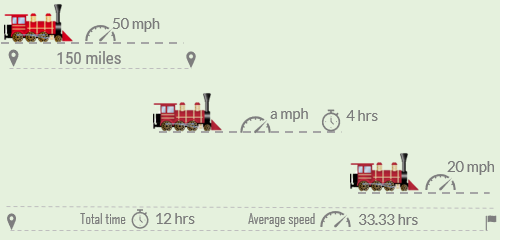
Solution
In this question, the journey is separated into 3 parts, with each of them having different speeds. We can consider the whole information in the following tabular form:
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 150 | 50 | 3 |
| 2nd part of the journey | a | 4 | |
| 3rd part of the journey | 100 | 20 | |
| Total | 12 |
As the total journey time is 12 hrs, the journey time for the 3rd part = 12 – (3 + 4) hrs = 5 hrs
Also, we know average speed = Total distance traveled/ Total time taken
Or, 33.33 = Total distance travelled/12
Or, Total Distance travelled = 33.33×12 = 400 miles
Therefore, the distance traveled in the 2nd part of the journey = 400 – (150 + 100) miles = 150 miles
So, the average speed in the 2nd part of the journey = 150/4 = 37.5 mph
Key Takeaways
In this question, we can see the application of average speed where the journey is completed in more than two parts. But the procedural approach of the concept remains same –
- Average speed is used as per the basic definition, i.e. the ratio of total distance traveled, and total time taken
- Whether the journey is divided into two or more than two parts, the procedure of calculating the average speed remains the same
Key Takeaways from the article | GMAT Word Problems | Time, Speed, and Distance
- By definition, the average speed of a journey = (total distance traveled)/(total time taken to travel that distance)
- If a journey is divided into two equal parts, with individual speeds a and b respectively, then the average speed of the whole journey is denoted by 2ab/(a+b). However, this formula cannot be generalized for a journey which is divided into more than 2 parts
- If a journey is divided into multiple parts, and the time taken to cover every part is same, then the average speed of the whole journey is denoted by the average or arithmetic mean of the individual speeds at which the individual parts are covered.
Questions on time, speed and distance are very commonly asked on the GMAT. Ace GMAT Quant by signing up for our free trial and get access to 400+ questions. We are the most reviewed online GMAT Prep company with 2400+ reviews on GMATClub.