A 7 min read

On the GMAT, Factorial is an interesting concept in number properties from which many application-oriented questions are asked. In this article, we will discuss one of the most frequently asked question types in factorials.

Objective of this Article

The primary objective of this article is to:

• Demonstrate 2 different cases in which the concept of factorials can be applied to find the correct answer.

To explains the 2 scenarios, we have taken the help of 3 questions. The first 2 questions will be used to explain Case 1 and the third question will be used to explain Case 2.

Let’s start with the first scenario.

Case 1:

The Context

Many times, we get questions on factorials where we need to find the highest power of a number present in the factorial. Sometimes, we also get questions where we need to find the number of trailing zeroes of a specific factorial value.

We will discuss both these cases one after the other and finally, relate them.

Question 1

The number n is obtained by multiplying the first 40 natural numbers. What is the value of the greatest integer p, for which 5^p is a factor of n?

1. 7
2. 8
3. 9
4. 10
5. 11

Understanding the Question

Let us read the question stem first and draw all the pertinent inferences from the given information.

In this question, we are given that n is a number which is obtained by multiplying the first 40 natural numbers.

• Now, when we multiply all the positive integers, starting from 1 to 40, both inclusive, we get 40!
• Hence, we can say n = 40!

So, re-framing the question, we can say that we need to find the greatest integer p, for which 5^p is a factor of 40!

• Doesn’t that also mean p is the highest power of 5 in the number 40!?
  • Yes, this is also another interpretation of the same statement.

Approach & Solution

So now, let’s find the highest power of 5 in 40! by dividing 40 with 5 and its consecutive higher powers till it is possible to divide, and then add the quotients of all the divisions, to determine our answer.

At this point, some of you may wonder why are dividing 40 by 5¹ and 5² only? Let’s figure it out.

• In the number 40!, we have different powers of 5. For example, some numbers are divisible by 5¹ whereas we have number which is divisible by 5²
• Now, we know that the number 5 occurs in every five consecutive numbers. So, if we move from 1 to 40, we can find the instances of 5, by simply dividing 40 by 5.
• Similarly, we get an instance of 25 in every 25 consecutive numbers. So, if we move from 1 to 40, we can find the instances of 25, by dividing 40 by 25.
• Now, do we need to check for the other powers of 5?
  • 5³ is 125, and in 40, we do not get an instance of 125. Hence, we do not need to check for 5³ or further powers of 5.

So, now let’s divide 40 by 5¹ and 5², to determine their individual instances.

• 40/5¹ = 8
• 40/5² = 40/25 = 1

5¹ and 5² are the only two powers of 5 that can divide 40. Higher powers of 5 (for example, 5³ = 125, or 5⁴ = 625 etc.) are greater than 40, hence we will not consider them while dividing with powers of 5.

Therefore, the highest power of 5 in 40! = 8 + 1 = 9

In other words, we can also say that the greatest value of p, for which 5^p is a factor of 40! Is 9.

So, the correct answer choice is option C.

Questions on Number Properties are very commonly asked on the GMAT. Ace GMAT Quant by signing up for our free trial and get access to 400+ questions. We are the most reviewed online GMAT Prep company with 2400+ reviews on GMATClub.

Save 60+ hours of GMAT preparation by crafting a well-defined study plan in just 3 steps:

Now, let’s solve one more similar question, to solidify our understanding.

Question 2

What is the highest power of 8, that can divide 88!?

1. 11
2. 12
3. 25
4. 28
5. 29

Understanding the Question

This question is similar to the previous question that we solved a while ago. Hence, you may think that we can apply the same method here.

Let’s see what happens when we do that.

In this question, we are asked to find the highest power of 8 that can divide the number 88!

So, we must divide 88 by powers of 8, till division is possible, and then add the quotients to obtain the highest power of 8.

• 88/8¹  = 11
• 88/8²  = 88/64 = 1

As 8³ = 512 is greater than 88, we don’t need to consider that.

Hence, the highest power of 8 that can divide 88! = 11 + 1 = 12

So, the correct answer choice is option B, right?

• No, 12 is not the correct answer.

Let’s analyse the question a bit more to understand the error.

Approach & Solution

When we divide 88! by 8 or powers of 8, we are only identifying and counting those numbers which are multiples of 8, such as 24, 32, 56 etc.

But, are these numbers the only ones by which we can get as a multiple of 8?

• Can we not get a multiple of 8 by multiplying a multiple of 2 and another multiple of 4?
  • Answer yourself.
• Let’s consider two numbers – 44 and 70 – where none of them are multiples of 8. Note that both these numbers are present in 88!
• Now 44 is a multiple of 4 and 70 is a multiple of 2, hence, their product must be a multiple of 4 x 2 = 8, right?
  • But when we divide 88 by the powers of 8, we didn’t consider these numbers!
  • Hence, just by dividing the number by 8, we may not get all such instances where we can get an 8 or its multiple.

In such cases, what should we do? We need to first express the number in the prime factorized form.

• We know 8 = 2 x 2 x 2 = 2³
• Hence, if we get three 2’s, then we can form an 8 from them. So, let’s find out the number of 2’s first.
  • 88/2¹ + 88/2²  + 88/2³  + 88/2⁴  + 88/2⁵  + 88/2⁶  = 44 + 22 + 11 + 5 + 2 + 1 = 85
  • Therefore, in 88! we have total 85 instances of 2.
  • From these 85 instances of 2, we can make 85/3 = 28 number of 8’s. (As 8 = 2³, to form an 8, we need 3 instances of 2)
  • Therefore, the highest power of 8 that can divide is 88! is 28. And the correct answer is option D.

Difference between Question 1 and Question 2

Well, we need to understand the fundamental difference between the two questions.

• In question 1, we directly divided the number by 5 to get the instances of 5 in the factorial value. Whereas in question 2, we first prime factorized 8 and then calculated the instances of the prime factor in the factorial value.

Whenever we need to calculate the instances of any prime number in a factorial value, we can do that by directly dividing the factorial number by the prime number. But, if we have a composite number, then first we need to express the number in terms of its prime factors. We need to prime factorize because apart from the number and its powers, we can get the instances of the number from the combination of other numbers also. (For example, we can get 8 by 4 x 2 or 2 x 2 x 2, apart from 8 or powers of 8)

Once we get the prime factor(s) of the number, we need to find the instances of each of the prime factor(s), and then from them, the instances of the original number.

Now, let’s solve another variation of the similar concept, on the applications of factorials.

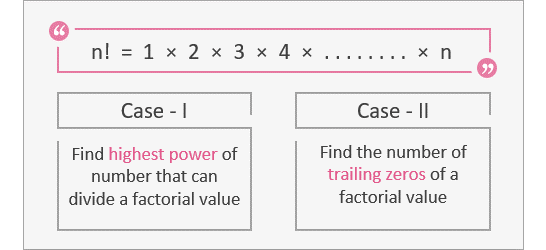
Case 2:

The context

Sometimes we get questions where we are given a factorial number, and we need to find the number of consecutive zeroes at the end of the value of the number, before the first non-zero digit from the right. This is another interesting application of the same concept of factorials that we discussed in the previous two questions. Let’s demonstrate that with an example.

Question 3

How many trailing zeroes would be found in 57! upon expansion?

1. 5
2. 13
3. 28
4. 53
5. 57

Understanding the Question

In this question, we are given a number 57! and we are asked to find the number of trailing 0s at the end of the value of 57!

The value of 57! is effectively a number which we get by multiplying all the positive integers from 1 to 57. But how do get 0s at the end of a product of two or more numbers?

• We get 0’s when we have presence of 10 or multiples of 10 in that product.
  • Hence, to find the number of trailing 0’s, we need to know the instances of 10 in 57!
  • Or in other words, we need to find the highest power of 10 that can divide 57!

Now, this is a known application to us – in the previous question, we solved the same only. So, let’s follow the same approach.

Approach & Solution

As 10 is a composite number, first we need to express it in terms of its prime factorized form.

• As 10 = 2¹ x 5¹, we need to find the instances of 2 and 5 separately in 57! and then we can calculate the number of possible pairs of 2 and 5, to get the instances of 10.
• Instances of 2: 57/2¹  + 57/2²  + 57/2³  + 57/2⁴  + 57/2⁵  =  57/2 + 57/4 + 57/8 + 57/16 + 57/32 = 28 + 14 + 7 + 3 + 1 = 53
• Similarly, instances of 5: 57/5¹  + 57/5²  = 57/5 + 57/25 = 11 + 2 = 13

Now, we have 53 instances of 2’s and 13 instances of 5’s. We also know that, to form a 10, we need 1 instance of 2 and 1 instance of 5.

Hence, number of possible pairs of 2 and 5 = minimum (53, 13) = 13

Or, we can also say that the number of trailing 0’s in 57! is 13.

Hence, the correct answer choice is option B.

Final Takeaways from the Article

• If we are asked to find the highest power of a number ‘n’ in a factorial, where n is a prime number, we can directly divide the factorial number by ‘n’ (and possible powers of ‘n’) to find the instances of ‘n’, and subsequently the highest power of ‘n’.
  • In case n is a composite number, we must express ‘n’ first in the prime factorized form. From the prime factorized form, we need to find the instances of all individual prime factors, and finally instances of ‘n’.
• If we are asked to find the number of trailing zeroes in a factorial value, essentially, we are asked to find the highest power of 10 that can divide the factorial value. In such case, we can express 10 as 2 x 5, and find the individual instances of 2 and 5 respectively. Finally, we can find out how many possible pairs of 2 and 5 can be created, to calculate the highest power of 10.