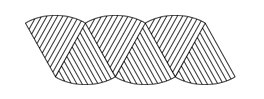
The figure above is constructed by separating a circular region into 6 equal parts and rearranging the parts as shown. If the diameter of the circle is d, what is the perimeter of the figure above?
| Source | OGQR 2020 |
| Type | Problem Solving |
| Topic | Geometry |
| Sub-Topic | Circle |
| Difficulty | Medium – Hard |
Solution
Given
In this question, we are given
- A diagram, which is constructed by separating a circular region into 6 equal parts and rearranging them, as shown in the diagram.
- The diameter of the circle is d.
To Find
We need to determine
- The perimeter of the figure, as shown.
Approach & Working
The perimeter of the given figure consists of 2 parts:
- 6 equal length arcs, which together form the perimeter of the circle
- 2 equal line segments, each of which is equal to the radius of the circle
The total length of the 6 arcs = the perimeter of the circle = 2π * (d/ 2) = πd
The total length of the 2 line segments = (d/ 2) + (d/ 2) = d
- Therefore, the perimeter of the given figure = πd + d
Hence, the correct answer is option D.
If you are planning to take the GMAT, we can help you with a personalized study plan and give you access to quality online content to prepare. Write to us at acethegmat@e-gmat.com. We are the most reviewed GMAT prep company on gmatclub with more than 2400 reviews and are the only prep company that has delivered more than 700+ scores than any other GMAT club partner. Why don’t you take a free trial and judge for yourself?










