OG 2020: Question No. 300
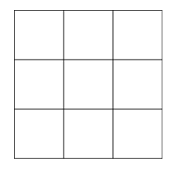
The 9 squares above are to be filled with x’s and o’s, with only one symbol in each square. How many of the squares will contain an x?
- More than ½ of the number of squares will contain an o.
- Each of the 4 corner squares will contain an x.
| Source | OG 2020 |
| Type | Data Sufficiency |
| Topic | Number Properties |
| Sub-Topic | Basic |
| Difficulty | Easy – Medium |
Solution
Steps 1 & 2: Understand Question and Draw Inferences
In this question, we are given
- A diagram, consisting of 9 squares.
- Each square is to be filled with x’s or o’s.
- There will be only one symbol in each square.
We need to determine
- The number of squares which will contain an x.
As there are total 9 squares, we need to know how many squares will have o’s, to determine the number of squares contains x’s.
With this understanding, let us analyse the individual statements.
Step 3: Analyse Statement 1
As per the information given in statement 1, more than half of the number of squares will contain an o.
- From this statement, we can conclude that at least 5 squares will contain an o, and therefore, at most 4 squares will contain a x.
- However, we cannot determine the exact number of squares that will contain an x.
Hence, statement 1 is not sufficient to answer the question.
Step 4: Analyse Statement 2
As per the information given in statement 2, each of the 4 corner squares will contain an x.
- From this statement, we cannot determine that how many of the remaining 5 non-corner squares will contain an x.
Hence, statement 2 is not sufficient to answer the question.
Step 5: Combine Both Statements Together (If Needed)
From statements 1 and 2 combined, we can say
- At most 4 squares will contain an x.
- Also, the 4 corner squares will contain an x.
Combining both information, we can conclude that there will be exactly 4 squares which will contain an x.
Hence, the correct answer choice is option C.














