A 4 min read

Want to test your grasp over GMAT Number Properties? Try this 700 level question!

If P and Q are positive integers and P = 3 + 2Q, find the GCD of P and Q.

 

(1)  P = 3x, where x is a positive integer

(2)  Q = 3y, where y is a positive integer

 

1. Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient to answer the question asked.
2. Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient to answer the question asked.
3. BOTH statements (1) and (2) TOGETHER are sufficient to answer the question asked, but NEITHER statement ALONE is sufficient to answer the question asked.
4. EACH statement ALONE is sufficient to answer the question asked.
5. Statements (1) and (2) TOGETHER are NOT sufficient to answer the question asked, and additional data specific to the problem are needed.

 

Did you get this question right? Good Job! You did better than most GMAT aspirants! And, if you didn’t, then read on to figure where you went wrong.

 

You should solve any Data Sufficiency question using the 5- Step Process we delineate below. That ways, you’ll never make any of the common mistakes that students commit while solving DS questions.

 

Steps 1 and 2: Understand the question and draw inferences

The question statement tells us the following pieces of information:

(i)              Both P and Q are positive integers

(ii)            P = 2Q + 3

 

So, this means that:

(i)              P is always odd (P is the sum of an odd and an even number)

(ii)            P is always greater than Q

(iii)           We can also write Q = (P – 3)/2

 

 

We need to find the GCD of P and Q.

 

Step 3: Analyse Statement 1 independently

 

Statement 1 tells us that:

P = 3x, where x is a positive integer

 

This means

2Q + 3 = 3x

• Q = 3(x – 1)/2

Since it is given that Q is an integer, we can safely conclude that (x – 1)/2 must be an integer.

 

In other words, (x – 1) must be an even number

• x must be an odd number (≠1 since Q is a positive integer and so Q > 0)

So let us take some values of x and see the corresponding values of P and Q.

 

 

 

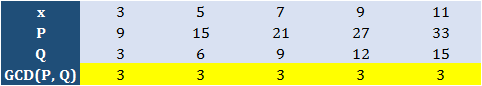
You can see that irrespective of the value of x (any odd integer), the GCD of P and Q is always 3.

 

 

An alternate way to look at things:

 

Observe the forms of P and Q carefully:

P = 3x and Q = 3(x – 1)/2

 

Since both P and Q are integers, we can safely conclude from the above that

1. Both P and Q are divisible by 3
2. (x – 1)/2 is an integer.

Let’s say (x – 1)/2 = k, where k is an integer.

• x = 2k + 1

Since we already know that P and Q are divisible by 3, it is enough if we find the GCD of x and (x – 1)/2 and then multiply it by 3 to get the GCD of P and Q.

• we need to find the GCD of k and 2k + 1

However, we know that the GCD of k and 2k + 1 is obviously equal to 1.

 

(2k and 2k + 1 do not have any common factors other than 1 since they are consecutive integers. Since k is a divisor of 2k, we can safely conclude that k and 2k + 1 also do not have any common factors other than 1)

 

Therefore the GCD of P and Q = 3 X 1 = 3.

 

Thus, Statement 1 is sufficient to find the GCD of P and Q.

 

Step 4: Analyse Statement 2 independently

Statement 2 tells us that:

 

Q = 3y

• P = 2(3y) + 3
• P = 3(2y + 1)

Therefore P = 3(2y + 1) and Q = 3y.

 

 

Notice that these two numbers are again in the same form as noticed in the Alternate explanation for Statement 1. (Observe the below steps)

1. P and Q are obviously divisible by 3.
2. Therefore GCD of P and Q is (GCD of y and 2y + 1)x3
3. However, GCD of y and 2y + 1 is 1.
4. Therefore GCD of P and Q is 1×3 = 3.

Therefore statement 2 alone is sufficient to arrive at a unique answer.

 

 

We can also approach this in the conventional method by taking values for y and calculating the GCD of P and Q.

 

 

 

 

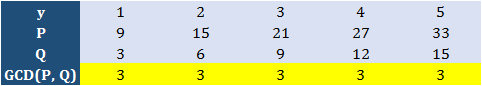
Notice that the GCD of P and Q is always 3 irrespective of the value of y.

 

Thus, Statement 2 alone is sufficient to arrive at a unique value of p.

 

Step 5: Analyse both statements together (if required)

We do this step only in cases where neither Step 3 nor Step 4 have given us a unique solution. In this question, we arrived at a unique solution in Step 3 and Step 4 individually. So, we do not need to do Step 5.

 

Answer

Thus, we see that the correct answer choice for this question is D – EACH statement ALONE is sufficient to answer the question asked.

 

 

 

Very frequently, you encounter questions on the GMAT that seem to be confusing and lengthy to solve. This is a trap to waste the test taker’s time. Test takers who are in a hurry to solve the question might miss the most obvious and elegant way to solve the question and thereby end up wasting their precious time.

 

So, when you start to solve a question, take a step back, carefully note down the given information and analyse it.

 

Most of the questions on the GMAT can be solved very easily and elegantly when one sticks to the basics without doing any careless mistakes.

 

Why limit your learning to only this one takeaway? A whole session of exciting GMAT 700+ level questions, deadly traps and “Aha!” takeaways awaits you this Saturday.

• Register for eGMAT’s Free Session on Number Properties here
• For those who have already registered, try out the free Number properties concept in our free trial.