OGQR 2020: Question No. 95
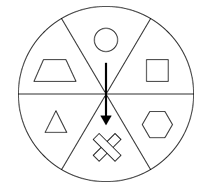
The figure above, which is divided into 6 sectors of equal area, contains an arrow representing a spinner. If the spinner is rotated 3,840 degrees in a clockwise direction from the position shown, which of the following indicates the sector to which the arrow on the spinner will point?
| Source | OGQR 2020 |
| Type | Problem Solving |
| Topic | Geometry |
| Sub-Topic | Circle |
| Difficulty | Medium |
Solution
Given
In this question, we are given
- The figure of a circle, divided into 6 sectors of equal area, contains an arrow representing a spinner.
- The spinner is rotated 3840 degrees in a clockwise direction from the position shown.
To Find
We need to determine
- Which of the given diagrams indicates the sector to which the arrow on the spinner will point.
Approach & Working
We know that for a complete revolution of 360 degrees, the pointer will return at the starting point.
- 3840 = 360 * 10 + 240
- Hence, after completing 10 complete revolutions, the pointer will move for another 240 degrees clockwise.
As the circular region is divided into 6 regions of equal area, each region is equivalent to 60 degrees.
Therefore, to make a movement of 240 degrees, the pointer will shift 4 more regions, and end up showing at square.
Hence, the correct answer is option B.














