Before you start reading this article on GMAT Number Properties : LCM – GCD, ask yourselves the following question:
a) Are you among those who wish that they do not encounter a LCM-GCD question on their exam day or
b) Are you among those who take a guess whenever they come across a LCM-GCD question or
c) Are you among those who try their mighty hard but still fall short of the correct answer in a LCM-GCD question
If yes, this article was waiting for you the moment you thought of taking the GMAT.
Here is a snapshot of the topics we’ll talk about in this article
- 3 primary pitfalls in the concept of LCM – GCD | GMAT Number Properties
- 5 GMAT Practice questions on LCM – GCD concept | GMAT Number Properties
- Takeaways
We at e-GMAT believe in making GMAT ‘easy’ for you. Based on our extensive interaction with more than 2000+ students spread over the live sessions and forum queries we have identified these three primary pitfalls in the concepts of LCM-GCD:
- Getting confused by different names of LCM & GCD
- Not having prime factorization as the default approach
- Being unmindful of constraints on LCM & GCD
In this article, we will explain the pitfalls in LCM-GCD questions along with the measures to overcome these pitfalls to help you ace 700+ questions of the topic. Let’s begin with the first and the most common pitfall.
1. Getting confused by different names of LCM & GCD | GMAT Number Properties
LCM-GCD are defined in various terms and phrases in questions which appear in GMAT. If you are not able to decipher the hidden meaning behind these terms you are likely to waste your precious time and even worse may approach the question with a disastrous approach. Thus, it is of utmost importance to know when the question is talking about LCM-GCD. Let’s see the ways in which a question can encrypt the message of LCM-GCD.
GCD can be expressed as the following terms/phrases:

a) x is the GCD of 12 & 18
b) x is the HCF of 12 & 18 (HCF stands for Highest Common Factor)
c) x is the highest number which divides both 12 & 18
All the 3 terms above refer to the GCD of 12 & 18 i.e. 6. Try focusing on keywords such as ‘Highest’, ‘Greatest’. These words are an indication of the question talking about the GCD of a set of numbers in a LCM-GCD question.
Let’s see now the terms/phrases which are used to express LCM:
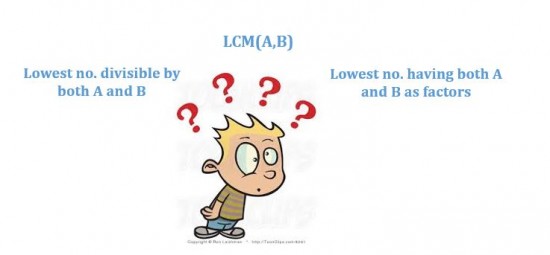
a) z is the LCM of 12 & 18
b) z is the lowest number which is divisible by both 12 & 18
c) z is the lowest number which has 12 & 18 as its factors
All the above terms refer to the LCM of 12 & 18 i.e. 36. Try focusing on keyword ‘lowest’ which can be an indication of question talking about LCM of a set of numbers.
The important point to remember for identification of both LCM & GCD is the definition of these terms. While GCD is the factor/divisor of a set of numbers, LCM is the multiple of a set of numbers. Whenever you are presented with a phrase which confuses you between LCM-GCD, try to breakdown the statement in the terms of factors and multiples. For example, refer the following GMAT practice questions:
Q1: Two positive integers a and b are divisible by 5, which is their largest common factor. What is the value of a and b?
I. The lowest number that has both integers a and b as its factors is the product of one of the integers and the greatest common divisor of the two integers.
II. The smaller integer is divisible by 4 numbers and has the smallest odd prime number as its factor.
The question plays with words when talking about LCM-GCD. Try answering this question by deciphering the message of LCM-GCD hidden in it. You can access the detailed solution here.
Takeaway
I. LCM of a set of numbers is divisible by all the numbers in the set
II. GCD of a set of numbers divides all the numbers in the set
Keeping these two definitions in mind and your heart will always keep you in good stead whenever you face a LCM-GCD question.
2. Not having prime factorization as the default approach | GMAT Number Properties
Many of the students at some time or the other have avoided doing prime factorization of a set of numbers for finding their LCM & GCD. Such an approach towards a LCM-GCD question most often leads to incorrect solution costing you precious marks and time 😥
We will explain you here, why and how prime factorization is a robust method in LCM-GCD questions and the potential pitfalls which you may avoid on your journey to a perfect quant score.

Let’s look at the following official GMAT Practice question:
Q2: If n is a positive integer, is n a multiple of 24?
I. n is a multiple of 4
II. n is a multiple of 6
The most common fallacy committed by students in such a question is to express n as a multiple of 4 & 6 i.e. 4x and 6y respectively and write n = 24xy. With this they conclude that n is a multiple of 24 which is an incorrect analysis.
Students who get it right are the ones who prime factorize 4 & 6 and find that
I. n is a multiple of 22 – so, n is a number of the form 22*k
II. n is a multiple of 2*3 – so, n is a number of the form 2*3m
The key here is to observe that n can be expressed as a multiple of maximum of 22 and as a multiple of maximum of 31. Combining these two, we can say that n can be expressed as a multiple of 22 * 3 i.e. 12. Hence n is a multiple of 12 and not 24. Thus it can be seen that the method of prime factorization helps in eliminating such mistakes in the approach of the students.
The other and the most significant benefit of prime factorization is to help you remember an easy mnemonic for calculating LCM & GCD. For calculating the GCD (Greatest Common Divisor), use the lowest power of primes of the set of numbers and for calculatingthe LCM (Least Common Multiple) use highest power of primesof the set of numbers. It’s a golden approach where you are never going to commit an error while calculating LCM-GCD of a set of numbers. Also, this approach is particularly helpful when calculating LCM-GCD of more than 2 numbers where the division method usually fails or takes up a lot of time.
Let’s understand another advantage of using prime factorization in LCM-GCD question with an example:
Q3: If the LCM of 36 & x is 72, what are the possible values of x?
At the first look, the question seems to have insufficient information provided. Let’s see if the prime factorization method leads to an answer.
It’s given that 72 is the LCM of 36 & x. Prime factorizing 72 would give us 72 = 23 * 32
Similarly 36 can be prime factorized as 36 = 22 * 32. Now, we know that for calculating LCM we take the highest power of primes. Since 72 has 32 in it x can have either {30,, 31 , 32}. Similarly x will have 23 in it as 36 has only 22 in it and the LCM has 23 in it. Now, x can’t have any more prime factor other than 2 or 3 as the LCM does not have any other prime factor apart from 2 and 3. So, possible values of x = {23 * 30, 23 * 31, 23 * 32} = {8, 24, 72}.
Thus, you see that prime factorization leads you out of a situation where the information seemed insufficient at the very first instant.
TAKEAWAY
I. Use Prime Factorization as your first line of offense against LCM-GCD questions.
3. Being unmindful of constraints on LCM & GCD
A few questions on GMAT test your understanding of the possible values of LCM & GCD of a set of integers. For example, have a look at the following official question( OG 13 QR2, Problem Solving QNo: 98):
Q4: Which of the following CANNOT be the greatest common divisor of two positive integers x and y?
(A) 1
(B) x
(C) y
(D) x -y
(E) x + y
The above question directly tests your knowledge of the range of LCM & GCD. Students not aware of the range of GCD would have trouble solving this question in the given time frame. Here is the solution to this GMAT practice question.
Let’s see & observe how we can find the range of LCM & GCD and how it can be used for solving questions on LCM-GCD.
GCD
As we know, GCD of a set of integers is the factor of all the integers in the set. Now, factor of an integer can’t be greater than the magnitude of the integer itself. Thus, the GCD of a set of integers can’t be greater than the magnitude of the smallest integer of the set.
GCD of a set of integers < = Magnitude of the smallest integer of the set
Also, we know that 1 is the factor of all integers. Thus, every integer will have at least one common factor i.e. 1. So, for integers which do not have any common prime factors, their only and hence their highest common factor would be 1.
GCD of a set of integers => 1
From the above two analysis we can conclude that
1 < = GCD of a set of integers < = Magnitude of the smallest integer of the set
Let’s see the range of LCM now.
LCM
As we know, LCM of a set of integers is the multiple of all the integers in the set. Now, the multiple of an integer can’t be smaller than the magnitude of the integer itself. Hence, common multiple of a set of integers can’t be smaller than the magnitude of the largest integer of the set.
LCM of a set of integers => Magnitude of the largest integer of the set
For finding the upper range of LCM of a set of integers let’s assume two integers
P = ax * by and Q = cz where a, b, c are three distinct prime numbers. As we know that for calculating LCM we take the highest powers of the prime numbers, so LCM (P, Q) = ax * by * cz = P * Q.
LCM of a set of integers <= Product of magnitude of all integers in the set
From the above two analysis we can conclude that,
Magnitude of the largest integer of the set <= LCM of a set of integers<= Product of magnitude of all integers in the set
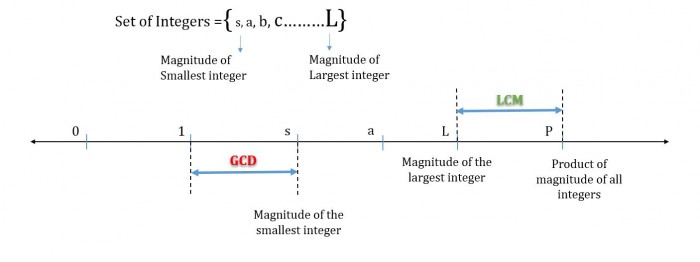
From the above interpretation and knowing that Product of all integers in the set = GCD * LCM, we can say that when LCM is equal to the product of all integers in the set, GCD = 1. We also remember that GCD = 1 when the integers of a set do not have any common prime factor. Thus we can say that for a set of integers which don’t have a common prime factor, GCD = 1 and LCM = Product of all integers on the set.
Given below are some practice questions which test your concepts of LCM-GCD
Q5: If A and B are positive integers greater than 1 such that the GCD of A and B is 1, then which of the following must be true?
I. A and B are prime numbers.
II. A and B are consecutive numbers.
III. A and B do not have a common prime factor
IV. The product AB has two prime factors
V. A and B have the opposite even-odd nature
(A) I and II only
(B) III only
(C) I, V only
(D) I,II, III, V only
(E) I, II, III, IV, V
Solution to Q5, of GMAT Number Properties : LCM – GCD
Q6: For two positive integers A & B, what is the highest number that divides completely the product of integers from 1 to A and 1 to B such that B = A + 29.
(A) 1
(B) Product of all integers from 1 to A
(C) Product of all integers from 1 to B
(D) 29*A
(E) Can’t be determined
Look at the solution of this GMAT practice question on number properties
Takeaway
I. 1 < = GCD of a set of integers < = Magnitude of the smallest integer of the set
II. Magnitude of the largest integer of the set <= LCM of a set of integers<= Product of magnitude of all integers in the set
Conclusion
We have observed from the above pitfalls that it’s easy to make LCM-GCD your friend. Since questions on LCM-GCD constitute an important part of GMAT Number Properties and are frequently tested on the test, it’s advisable to be proficient on this topic. Once you have gone through the above article and identified the potential pitfalls in LCM-GCD question, practice more questions on such concepts to prepare yourself better for the exam day.
Hope that this article would be a ‘factor’ in your quant preparation and your joy is a ‘multiple’ of your quant score.
Wish you a great GMAT journey
Other Relevant Articles
Here are the links to a few other articles which would help you strengthen your concepts on Number Properties:
3 mistakes in GMAT even-odd questions – An article which describes the common pitfalls students make in even-odd questions. Read it to become an even-odd champion














